(→Sample Results) |
(→Sample Results) |
||
| Line 16: | Line 16: | ||
|} | |} | ||
BC are Homogeneous Neumann and Non-Homogeneous Dirichlet. Each elementary cell is of the non-ideal (non-linear) type. | BC are Homogeneous Neumann and Non-Homogeneous Dirichlet. Each elementary cell is of the non-ideal (non-linear) type. | ||
| + | |||
| + | |||
| + | {| class="wikitable" | ||
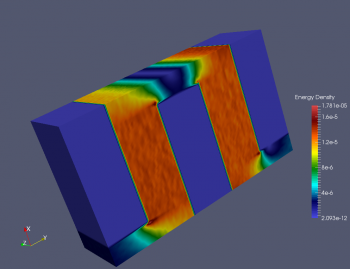
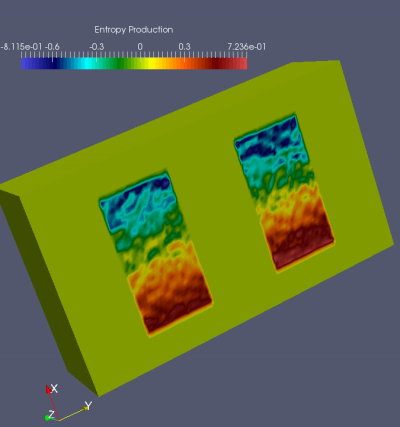
| + | |+ Sample 3D results for N-type junction with non-constant TE coefficients. | ||
| + | |[[File:OUIEnergyDensity3D.png|350 px]] | ||
| + | |[[File:OUIEntropyProduction.png|400 px]] | ||
| + | |} | ||
| + | |||
| + | |||
| + | |||
| + | BC are Homogeneous Neumann and/or Non-Homogeneous Dirichlet. Each elementary cell is of the non-ideal (non-linear) type. | ||
==Sub-modules for == | ==Sub-modules for == | ||
Revision as of 11:59, 1 April 2016
The DYCO Solvers suite
DYCO is a suite of solvers able to compute high accuracy solutions to non-linear
Main features
- Solving
Sample Results
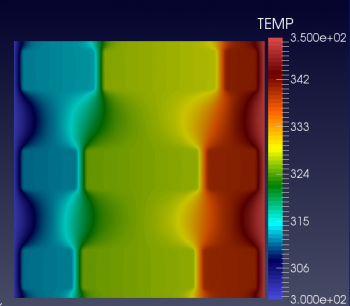
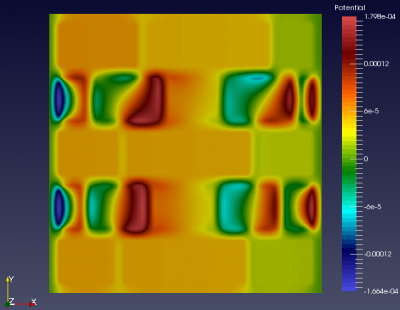
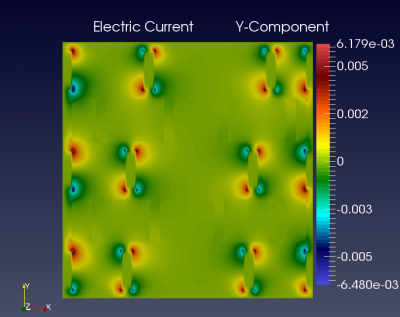
We show below a short gallery of pictures obtained using the DYCO solver, in the context of
|
|
|
BC are Homogeneous Neumann and Non-Homogeneous Dirichlet. Each elementary cell is of the non-ideal (non-linear) type.
|
|
BC are Homogeneous Neumann and/or Non-Homogeneous Dirichlet. Each elementary cell is of the non-ideal (non-linear) type.
Sub-modules for
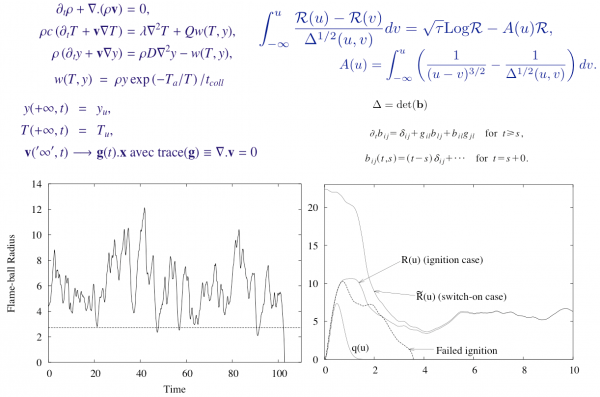
A sub-module of DYCO has been devoted to the numerical solution
|
Participants
Yves D'Angelo, Christophe Goupil, Eric Herbert, Xanthippi Zianni, Louise Meteir, Aurélie Louis-Napoléon.
