| Line 1: | Line 1: | ||
| − | The FLAMEX Solver | + | ==The FLAMEX Solver== |
[[File:Flamex.jpg|240px]] | [[File:Flamex.jpg|240px]] | ||
| − | + | FLAMEX is a suite of solvers able to compute high accuracy solutions to asymptotics-based evolution equations (EE). | |
| + | For propagatong fronts, the EEM (Evolution Equation Modeling) can be found by solving exactly the set of | ||
| + | * Euler equations | ||
| + | * Rankine-Hugoniot jump relations | ||
| + | * the local kinematic relation defining the local front velocity (e.g. with respect to local curvature). | ||
| + | when the density contrast between 'hot' and 'cold' fluids is asymptotically small. | ||
| + | |||
| + | |||
| + | {| class="wikitable" | ||
| + | |+ Examples of obtained EEM: for 2D planar, 3D planar, or 3D expanding fronts | ||
| + | |[[File:OUIEEM-1D-2D.png|400 px]] | ||
| + | |[[File:OUIEqEEM_1D.png|400 px]] | ||
| + | |} | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | == Main features == | ||
| + | |||
| + | * Solving first-order or second order EE in the Fourier or Fourier-Legendre basis. | ||
| + | * Time resolution using ETDRK1 and 4, using contour integrals to avoid cancelation errors (Trefethen et al. 2005) | ||
| + | * Laminar or turbulent configurations (EE with additive noise, e.g. Passot-Pouquet, Kraichnan-Celik, Von Karman/Pao or 'DNS turbulence') | ||
| + | |||
| + | |||
| + | |||
| + | ==Sub-module for asymptotic modeling of Flame-Balls== | ||
| + | |||
| + | A sub-module of FLAMEX has also been devoted to the | ||
| + | |||
| + | |||
| + | == Sample Results == | ||
| + | * See some comparisons between FLAMEX and DNS (HALLEGRO) results [http://www.coria-cfd.fr/index.php/H-Allegro#Direct_simulation_of_propagating_flames:_3D_expanding_front_.28Eric_Albin_.26_Yves_D.27Angelo.29] | ||
| + | * More details (and comparisons with experimental results) in | ||
Revision as of 10:56, 1 April 2016
Contents
The FLAMEX Solver
FLAMEX is a suite of solvers able to compute high accuracy solutions to asymptotics-based evolution equations (EE). For propagatong fronts, the EEM (Evolution Equation Modeling) can be found by solving exactly the set of
- Euler equations
- Rankine-Hugoniot jump relations
- the local kinematic relation defining the local front velocity (e.g. with respect to local curvature).
when the density contrast between 'hot' and 'cold' fluids is asymptotically small.
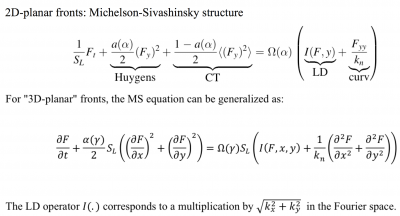
|
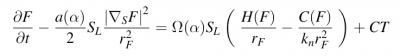
|
Main features
- Solving first-order or second order EE in the Fourier or Fourier-Legendre basis.
- Time resolution using ETDRK1 and 4, using contour integrals to avoid cancelation errors (Trefethen et al. 2005)
- Laminar or turbulent configurations (EE with additive noise, e.g. Passot-Pouquet, Kraichnan-Celik, Von Karman/Pao or 'DNS turbulence')
Sub-module for asymptotic modeling of Flame-Balls
A sub-module of FLAMEX has also been devoted to the
Sample Results
- See some comparisons between FLAMEX and DNS (HALLEGRO) results [1]
- More details (and comparisons with experimental results) in
