Revision as of 20:02, 3 April 2016

The DYCO Solvers suite

DYCO is a suite of solvers able to compute high accuracy solutions to non-linear stock/flow potentials coupled equations.

It is based on a nodal approach strategy.

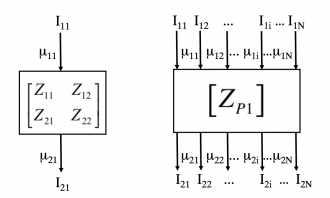
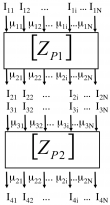
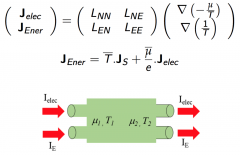
Left: Elementary Cell, Coupled potentials (1 or N coupled potentials, also in series)
Right: example in the thermo-electric context

Main features

• Nodal description of the considered network.
• Non linear Onsager type coupling between forces & fluxes.
• Steady, pseudo-unsteady & unsteady computations.
• Handle local to global scales (i.e. from coarse-grain to fine tuning).
• Possibly complex non-homogeneous structures and topologies.
• Possibly anisotropic, discontinuous coupling coefficients; potentials & time dependency can also be included.
• Local flux continuity enforced
• Allows for lighter/heavier computations and technological “optimization” !

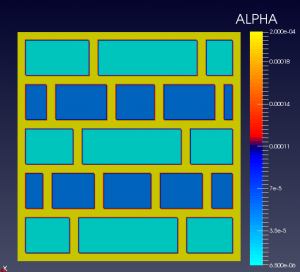
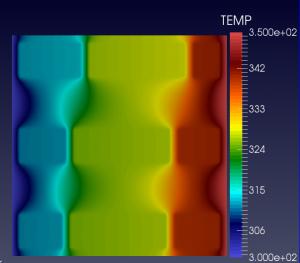
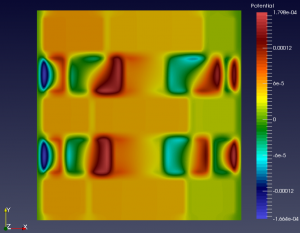
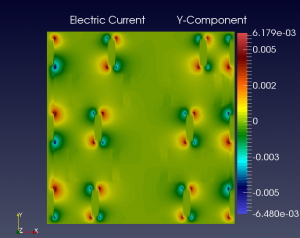
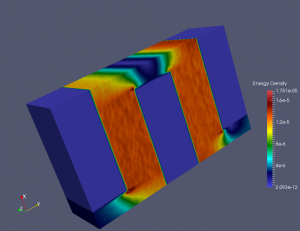
Sample Results

We show below a short gallery of pictures obtained using the DYCO solver, in the thermo-electric context.

Sample 3D results including a non-homogeneous thermo-electric material with non-constant TE coefficients.

Sample 3D results including a non-homogeneous thermo-electric material with non-constant TE coefficients, continued.

Sample 3D results for N-type junction with non-constant noisy TE coefficients.

In both cases, BC are Homogeneous Neumann and/or Non-Homogeneous Dirichlet.
Each elementary cell is of the non-ideal (non-linear) type.

Sub-modules

More specific sub-modules of DYCO shall be devoted to the numerical solution of coupled stock/flow potentials dynamics in the ecological economics and biological contexts.

The numerical solution to the coupling between 1D/3D Maxwell and heat equations is also in progress.

Participants

Yves D'Angelo, Christophe Goupil, Eric Herbert, Xanthippi Zianni, Louise Méteier, Aurélie Louis-Napoléon.